How to Write a Unit Circle Easy
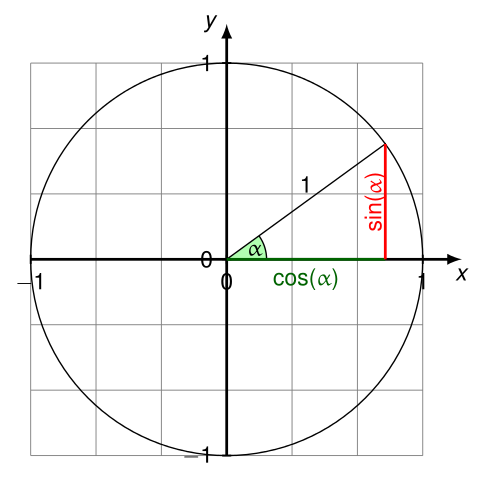
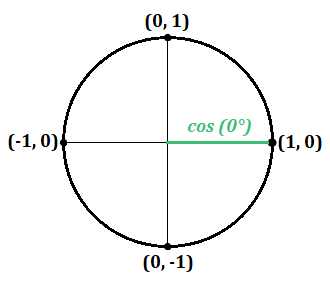
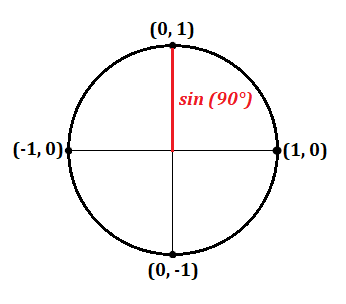
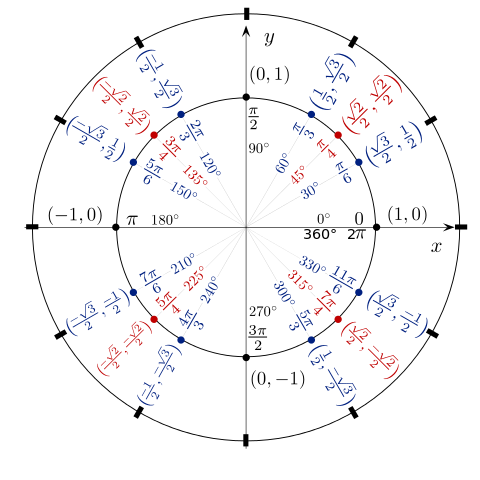
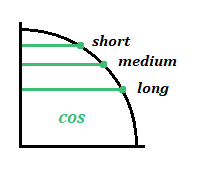
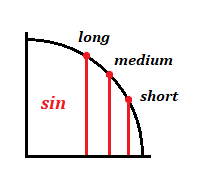
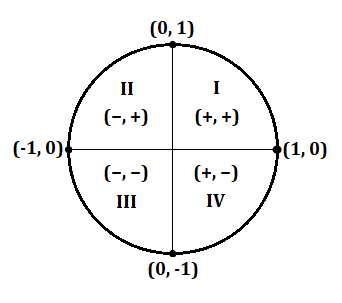
If you're studying trig or calculus—or getting ready to—you'll need to get familiar with the unit circle. The unit circle isan essential tool used to solve for the sine, cosine, and tangent of an angle. But how does it work? And what information do you need to know in order to use it? In this article, we explain what the unit circle is and why you should know it. We also give you three tips to help you remember how to use the unit circle. Feature Image: Gustavb/Wikimedia The unit circle is a circle with a radius of 1. This means that for any straight line drawn from the center point of the circle to any point along the edge of the circle, the length of that line will always equal 1. (This also means that the diameter of the circle will equal 2, since the diameter is equal to twice the length of the radius.) Typically, the center point of the unit circle is where the x-axis and y-axis intersect, or at the coordinates (0, 0): The unit circle, or trig circle as it's also known, is useful to know because it lets us easily calculate the cosine, sine, and tangent of any angle between 0° and 360° (or 0 and 2π radians). As you can see in the above diagram, by drawing a radius at any angle (marked by ∝ in the image), you will be creating a right triangle. On this triangle, the cosine is the horizontal line, and the sine is the vertical line. In other words,cosine = x-coordinate, and sine = y-coordinate. ( The triangle's longest line, or hypotenuse, is the radius and therefore equals 1.) Why is all of this important? Remember that you can solve for the lengths of the sides of a triangle using the Pythagorean theorem, or $a^2+b^2=c^2$ (in which a and b are the lengths of the sides of the triangle, and c is the length of the hypotenuse). We know that the cosine of an angle is equal to the length of the horizontal line, the sine is equal to the length of the vertical line, and the hypotenuse is equal to 1. Therefore, we can say that the formula for any right triangle in the unit circle is as follows: $$\cos^2θ+\sin^2θ=1^2$$ Since $1^2=1$, we can simplify this equation like this: $$\cos^2θ+\sin^2θ=1$$ Be aware thatthese values can be negative depending on the angle formed and what quadrant the x- and y-coordinates fall in (I'll explain this in more detail later). Here is an overview of all major angles in degrees and radians on the unit circle: But what if there's no triangle formed? Let's look at what happens when the angle is 0°, creating a horizontal straight line along the x-axis: On this line, the x-coordinate equals 1 and the y-coordinate equals 0. We know that the cosine is equal to the x-coordinate, and the sine is equal to the y-coordinate, so we can write this: What if the angle is 90° and makes a perfectly vertical line along the y-axis? Here, we can see that the x-coordinate equals 0 and the y-coordinate equals 1. This gives us the following values for sine and cosine: As stated above, the unit circle is helpful because it allows us to easily solve for the sine, cosine, or tangent of any degree or radian. It's especially useful to know the unit circle chart if you need to solve for certain trig values for math homework or if you're preparing to study calculus. But how exactly can knowing the unit circle help you? Let's say you're given the following problem on a math test—and are not allowed to use a calculator to solve it: $$\sin30°$$ Where do you start? Let's take a look at the unit circle chart again—this time with all major angles (in both degrees and radians) and their corresponding coordinates: Don't get overwhelmed! Remember, all you're solving for is $\sin30°$. By looking at this chart, we can see that the y-coordinate is equal to $1/2$ at 30°. And since the y-coordinate equals sine, our answer is as follows: $$\sin30°=1/2$$ But what if you get a problem that uses radians instead of degrees? The process for solving it is still the same. Say, for example, you get a problem that looks like this: $$\cos{{3π}/4}$$ Again, using the chart above, we can see that the x-coordinate (or cosine) for ${3π}/4$ (which is equal to 135°) is $-{√2}/2$. Here's what our answer to this problem would look like then: $$\cos({3π}/4)=-{√2}/2$$ All of this is pretty easy if you have the unit circle chart above to use as a reference. But most (if not all) of the time, this won't be the case, and you'll be expected to answer these types of math questions using your brain only. So how can you remember the unit circle? Read on for our top tips! In this section, we give you our top tips for remembering the trig circle so you can use it with ease for any math problem that requires it. In order to use the unit circle effectively, you'll need to memorize the most common angles (in both degrees and radians) as well as their corresponding x- and y-coordinates. The diagram above is a helpful unit circle chart to look at, since it includes all major angles in both degrees and radians, in addition to their corresponding coordinate points along the x- and y-axes. Here is a chart listing this same information in table form: Angle (Degrees) Angle (Radians) Coordinates of Point on Circle 0° / 360° 0 / 2π (1, 0) 30° $π/6$ $({√3}/2, 1/2)$ 45° $π/4$ $({√2}/2, {√2}/2)$ 60° $π/3$ $(1/2,{√3}/2)$ 90° $π/2$ (0, 1) 120° ${2π}/3$ $(-1/2, {√3}/2)$ 135° ${3π}/4$ $(-{√2}/2, {√2}/2)$ 150° ${5π}/6$ $(-{√3}/2, 1/2)$ 180° π (-1, 0) 210° ${7}/6$ $(-{√3}/2, -1/2)$ 225° ${5π}/4$ $(-{√2}/2, -{√2}/2)$ 240° ${4π}/3$ $(-1/2, -{√3}/2)$ 270° ${3π}/2$ (0, -1) 300° ${5π}/3$ $(1/2, -{√3}/2)$ 315° ${7π}/4$ $({√2}/2, -{√2}/2)$ 330° ${11π}/6$ $({√3}/2, -1/2)$ Now, while you're more than welcome to try to memorize all these coordinates and angles, this is a lot of stuff to remember. Fortunately, there's a trick you can use to help you remember the most important parts of the unit circle. Look at the coordinates above and you'll notice a clear pattern: all points (excluding those at 0°, 90°, 270°, and 360°) alternate between just three values (whether positive or negative): Each value corresponds to a short, medium, or long line for both cosine and sine: Here's what these lengths mean: For example, if you're trying to solve $\cos{π/3}$, you should know right away that this angle (which is equal to 60°) indicates a short horizontal line on the unit circle. Therefore,its corresponding x-coordinate must equal $1/2$ (a positive value, since $π/3$ creates a point in the first quadrant of the coordinate system). Finally, while it's helpful to memorize all the angles in the table above, note that by far the most important angles to remember are the following: It's critical to be able to distinguish positive and negative x- and y-coordinates so that you're finding the correct value for a trig problem. As a reminder,w hether a coordinate on the unit circle will be positive or negative depends on which quadrant (I, II, III, or IV) the point falls under: Here's a chart showing whether a coordinate will be positive or negative based on the quadrant a particular angle (in degrees or radians) is in: Quadrant X-Coordinate (Cosine) Y-Coordinate (Sine) I + + II − + III − − IV + − For example, say you're given the following problem on a math test: $$\cos210°$$ Before you even try to solve it, you should be able to recognize that the answer will be a negative number since the angle 210° falls in quadrant III (where x-coordinates are always negative). Now, using the trick we learned in tip 1, you can figure out that an angle of 210° creates a long horizontal line. Therefore, our answer is as follows: $$\cos210°=-{√3}/2$$ Lastly, it's essential to know how to use all of this information about the trig circle and sine and cosine in order to be able to solve for the tangent of an angle. In trig, to find the tangent of an angle θ (in either degrees or radians), you simply divide the sine by the cosine: $$\tanθ={\sinθ}/{\cosθ}$$ For instance, say you're trying to answer this problem: $$\tan300°$$ The first step is to set up an equation in terms of sine and cosine: $$\tan300°={\sin300°}/{\cos300°}$$ Now, to solve for the tangent, we need to find the sine and cosine of 300°. You should be able to quickly recognize that the angle 300° falls in the fourth quadrant, meaning that the cosine, or x-coordinate, will be positive, and the sine, or y-coordinate, will be negative. You should also know right away that the angle 300° creates a short horizontal line and a long vertical line. Therefore, the cosine (the horizontal line) will equal $1/2$, and the sine (the vertical line) will equal $-{√3}/2$ (a negative y-value, since this point is in quadrant IV). Now, to find the tangent, all you do is plug in and solve: $$\tan300°={-{√3}/2}/{1/2}$$ $$\tan300°=-√3$$ Now that you know what the unit circle looks like and how to use it, let's test what you've learned with a few practice problems. With this problem, there are two pieces of information you should be able to identify right away: Since 45° indicates a positive, medium-length line, the correct answer is ${√2}/2$. If you're not sure how to figure this out, draw a diagram to help you determine whether the length of the line will be short, medium, or long. Like problem #1 above, there are two pieces of information you should be able to quickly grasp with this problem: Since 240° indicates a negative, short line, the correct answer is $-1/2$. Unlike the problems above, this problem uses radians instead of degrees. Although this might make the problem look trickier to solve, in reality it uses the same basic steps as the other two problems. First, you should recognize that the angle ${5π}/3$ is in quadrant IV, so the x-coordinate, or cosine, will be a positive number. You should also be able to tell that ${5π}/3$ creates a short horizontal line. This gives you enough information to determine that theanswer is $1/2$. This problem deals with tangent instead of sine or cosine, which means that it'll require a little more math on our end. First off, recall the basic formula for finding tangent: $$\tan θ={\sin θ}/{\cos θ}$$ Now, let's take the degree we've been given—${2π}/3$—and plug it into this equation: $$\tan {2π}/3={\sin {2π}/3}/{\cos {2π}/3}$$ You should now be able to solve for the sine and cosine separately using what you've memorized about the unit circle. Since the angle ${2π}/3$ is in quadrant II, the x-coordinate (or cosine) will be negative, and the y-coordinate (or sine) will be positive. Next, you should be able to determine based on the angle alone that the horizontal line is a short line, and the vertical line is a long line. This means that the cosine is equal to $-1/2$, and the sine is equal to ${√3}/2$. Now that we've figured out these values, all we have to do is plug them into our initial equation and solve for the tangent: $$\tan {2π}/3={{√3}/2}/{-1/2}$$ $$\tan {2π}/3=-√3$$ If you're taking the SAT or ACT soon, you'll need to know some trig so you can do well on the math section. Take a look at our expert guides to trig on the SAT and ACT so you can learn exactly what you'll need to know for test day! Besides memorizing the unit circle, it's a good idea to learn how to plug in numbers and how to plug in answers. Read our guides to learn all about these two useful strategies, which you can use on any math test—including the SAT and ACT!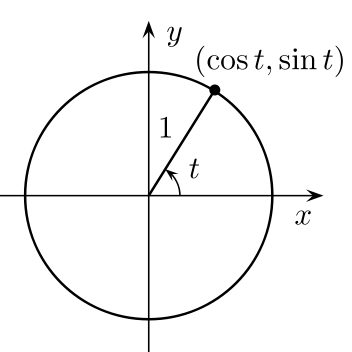
The Unit Circle: A Basic Introduction
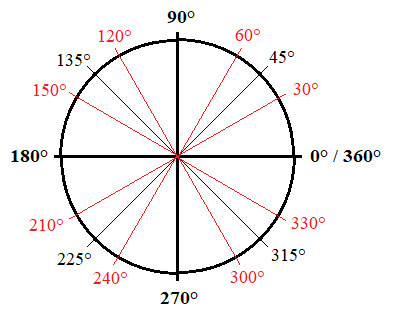
Unit Circle — Degrees
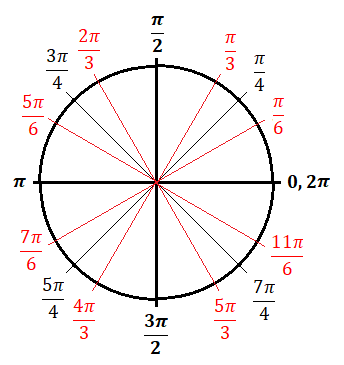
Unit Circle — Radians
 This slogan definitely applies if you're not a math lover.
This slogan definitely applies if you're not a math lover. Why You Should Know the Unit Circle
 Jim.belk/Wikimedia
Jim.belk/Wikimedia How to Remember the Unit Circle: 3 Essential Tips
 I wouldn't recommend practicing the unit circle with post-its, but, hey, it's a start.
I wouldn't recommend practicing the unit circle with post-its, but, hey, it's a start. #1: Memorize Common Angles and Coordinates
 Treat your negatives and positives as you would cables that can potentially kill you if hooked up incorrectly.
Treat your negatives and positives as you would cables that can potentially kill you if hooked up incorrectly. #2: Learn What's Negative and What's Positive
#3: Know How to Solve for Tangent
 Time to purr-actice your math skills!
Time to purr-actice your math skills! Unit Circle Practice Question Set
Questions
Answers
Answer Explanations
#1: $\sin45°$
#2: $\cos240°$
#3: $\cos{5π}/3$
#4: $\tan{2π}/3$
What's Next?

About the Author
Hannah received her MA in Japanese Studies from the University of Michigan and holds a bachelor's degree from the University of Southern California. From 2013 to 2015, she taught English in Japan via the JET Program. She is passionate about education, writing, and travel.
Source: https://blog.prepscholar.com/unit-circle-chart-radians-degrees
0 Response to "How to Write a Unit Circle Easy"
Post a Comment